Langrange, who shaped humanity and science, gained his passion for mathematics and astronomy after reading an article.
Born in Turin, Italy in 736, mathematician and astronomer Joseph-Louis Lagrange was not really interested in mathematics until the age of 17. His father wanted him to become a lawyer. Although he agreed to this as a child, he decided that he wanted to deal with positive sciences. At school, he read the works of Eucleides and Archimedes reluctantly, but the turning point in his life was when he read an article by the astronomer and mathematician Edmund Halley on the superiority of analysis over Greek mathematics.
- An Artificial Intelligence Created That Can Think Like Babies
- YouTube Creates Playlist of Videos with Over 1 Billion Views
Subsequently, he began to study mathematics so brilliantly that by the age of nineteen he had been appointed professor of mathematics at the Regie Scuole di Artiglieria in Turin. In 1755, Lagrange began working on some isoperimetric problems that Euler and some other mathematicians were trying to solve. The following year, using the analysis of variations in mechanics, he showed that this method offered a general procedure for solving dynamical problems in the form of the principle of least action. He shared these results with Euler. Euler had obtained similar results; however, he saw that Lagrange’s methods were more general and immediately wrote back to Lagrange, expressing his admiration for his ideas. These results laid the foundations of the branch of science that Euler would call the Calculus of Variations in the 1766s.
He subsequently published three important papers. The first was on the analysis of variations, the other on the application of the calculus of derivatives to probability theory. The third paper, which ended the difference of opinion between D’ Alembert and Euler in favor of Euler, provided a mathematical description of a vibrating wire.

In 18th century Europe, scientific academies encouraged research in celestial mechanics by offering prizes for the solution of specific problems. The main reason for this was that such research was especially useful for navigation. In 1764, Lagrange won the grand prize in a competition organized by the Academie Royale des Sciences in Paris to determine the gravitational forces that cause the Moon to almost always show the same face to the Earth. Two years later he was again awarded the grand prize, this time for a partial solution to a more complex problem concerning the gravitational forces between Jupiter, its four then-known moons and the Sun.
Towards the end of 1766, when Euler left Berlin, Frederik the Great said to Lagrange, “A great king like me is worthy of a great mathematician like you” and invited him to the Berlin Academy. Lagrange accepted this invitation and went to Berlin, where he would work for 20 years.
The Berlin years were very productive for his mathematical studies. Since he did not have to give lectures, he focused on his studies. In 1767, he published a treatise entitled “On the Solution of Numerical Equations”; four years later he presented another treatise entitled “Thoughts on the Algebraic Solution of Equations”. He solved some of the problems in number theory proposed by Fermat. He continued his work on interplanetary gravitational forces, winning the grand prize for the third time in 1772 for his study of the attraction between the Sun, Moon and Earth.
Lagrange’s method of working on a problem was slightly different from other mathematicians. Before starting a paper, he would solve the problem step by step in detail. When he finally wrote down his ideas, he rarely needed to make any corrections. Meticulous work and daring originality are the hallmarks of Lagrange’s mathematical publications.
During the twenty years he worked in Germany, Lagrange worked on his magnum opus, which he called Mechanique Analitique (Analytical Mechanics). It was about the application of analysis to solids. Around 1780, Lagrange became depressed and lost interest in mathematics in general for several years. “I feel my inertia increasing, and I don’t know if I will still be doing mathematics ten years from now,” he wrote to d’Alembert: “It seems to me that the mine is already very deep and must be abandoned unless new deposits are discovered.”
Lagrange became a member of the Paris Academy of Sciences in 1787. He spent the rest of his life in Paris. He lived through the French Revolution and managed to save his life in the revolution in which many of his contemporaries lost their lives. He was exempted by name from the decree ordering all foreigners to leave the country. Nevertheless, his depression did not abate. He told friends and colleagues that mathematics was no longer important to him.
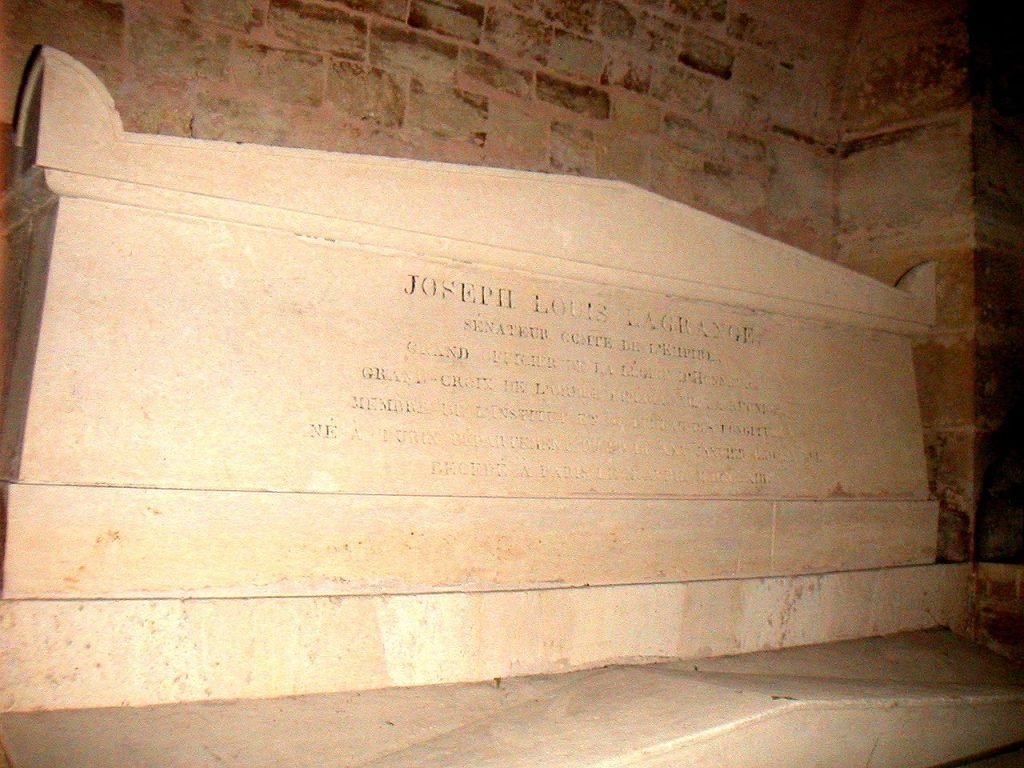
Lagrange died in Paris in 1813 after a short period of illness. He was buried in the Pantheon, near the tomb of Dekart. This genius, which France was proud to possess, became the honor of all humanity with his work. After his death, he left examples to follow, new problems to solve and methods to develop in all branches of mathematics. Bonaparte described him as the “supreme pyramid” of the mathematical sciences. Today, when physics students learn mechanics, they also learn Lagrangian mechanics, which describes the state of a dynamic physical system.